Introduction
Our Creator is the God of order and stability (Genesis 1:31 Deuteronomy 32:4, 1Samuel 2:2, Isaiah 26:4, Malachi 3:6, Matthew 7:24-27, Hebrews 13:8, James 1:17, et al. God being a “rock” signifies stability, strength, and unchangeability). Why then did He interject unstable equilibria into His creative handiwork? (If the reader wishes, but not necessary, see Equilibrium: chemistry, economics, mechanics.) When we use the term “interject” it assumes purposeful interjection—the interjections were purposed. But why? Bottom line, unstable equilibria in the created order serve the Creator’s overall goal of achieving functionality. That is, unstable equilibria play a crucial role in ensuring systems functionality in the created order. Sure, they might not always seem to serve that goal at first glance—but they definitely do—so, we must dig deeper, beyond first glances. To dig a little deeper is where we are heading, that is our goal in this study.
First some definitions. Equilibrium refers to a state of balance or stability. While it often relates to static situations (no net force/acceleration, e.g., a picture hanging undisturbed on a wall), it is not limited to static situations and can mean dynamic equilibrium. For example, orbital motion at constant speed; though there is centripetal acceleration in that motion, there is no radial acceleration, a centripetal force (mass times centripetal acceleration, this acceleration and hence force is “center seeking,” that is, directed inward) keeps the object steady in its orbit at a constant radius, i.e., it doesn’t “fly off” like a sling shot projectile. A centripetal force is essential for uniform circular motion, it ensures that the object’s speed remains constant while its velocity direction changes (speed is a scalar, velocity is a vector; velocity involves speed and direction; gravity, the tension in a slingshot string, etc., are examples of forces that provide manifest centripetal or simply put “center seeking” force). A simpler example of dynamic equilibrium is a car travelling straight ahead at constant speed. There are three kinds (states) of equilibrium: stable, unstable, and neutral; we will be focusing on the first two in this study. Stable equilibrium is such that when a system is displaced from equilibrium, it experiences a net force or torque opposite to the displacement causal (a restoring force). Most systems in the created order fall into this category. If a system, when displaced, experiences a net force or torque in the same direction as the displacement causal, then it is in unstable equilibrium. Even slightly induced displacements can cause acceleration away from equilibrium here. And when a system remains in equilibrium regardless of displacements from its original position, we have neutral equilibrium. When in neutral equilibrium, an object or body when displaced neither displaces further nor returns to its pre-disturbed position. For example, a marble on a flat surface may be slightly nudged and displaced one way or another without any major impact on the equilibrium—the marble largely stays where it is. The salient difference between stable and neutral equilibrium is that there is no restoring force at play in the neutral equilibrium case.
Downhill Flows Revisited
Potential (V), that is, potential energy per unit “something” (V=PE/unit something), is the key to understanding unstable equilibria. The created order was designed so that its systems lower their potential. The Creator’s systems are coded up to search for, to “sniff out” if you will, potential-lowering forces. Forces (F) are the dynamic that lower potential. That’s their job (F=-del[V]—note the minus sign; this literally says, forces drive potential down). Look if you will at the decidedly classical “W” gravitational PE curve below, it has a marble atop the middle PE maximum in unstable equilibrium (Fig 1). If we divide out the marble’s mass, we are looking at its gravitational potential curve V. (V is nice because looking at it allows one to readily compare the PE of objects of different mass at different heights.) The slightest disturbance impacting the marble will drive it to one or the other of the two bottom PE minima. These bottom minima are stable equilibria[1] (cf. “downhill flows”). That is an important takeaway about unstable equilibria—they serve as transition points leading to stability. Thus, they drive the Creator’s goal for dynamics. Given a small disturbance, systems tend to move away from unstable equilibrium toward stable equilibrium. In this way unstable equilibria betray system sensitivity to change. Looking at this classically, the disturbance simply acts as a trigger, the force F that actually lowers the marble’s potential (the F in F=-del[V]) is gravitational, and this gravitational force causes the marble to accelerate down the PE curve toward one or the other PE minima. Note, the disturbance is not the F in the equation, that F is gravitational; moreover, as said in the note, the marble’s total energy at any time is the sum of its potential and kinetic energy—we are largely interested in its PE component in this discussion going forward. General relativity (GR) paints a more profound picture of the action. GR tells us that gravity is not a force in the classical sense, rather, it stems from the curvature (gross distortion) of the “rubber sheet fabric” of spacetime around massive objects, where anything that carries energy, momentum, and stresses, as would a massive object, acts as a source of gravitational field—which corresponds to the curvature of spacetime (fields per se= force/unit mass or charge). In short, matter and energy encode, very precisely, spacetime curvature, and this curvature in turn affects the motion of objects within it. This is a classic rule-based negative feedback system seen again and again in the created order where the rules in this case guide spatial geometry updates per neighborhood mass-energy-stress content. (Before going any further, we should make clear that the “rubber sheet fabric” of spacetime mentioned above is a helpful visual aid, but no more.) Now, spacetime is not static, it is quite dynamic—there is a time dimension to spacetime as the word suggests. Objects experience (gravitational) time dilation near massive bodies due to these bodies’ gravitational influence. For example, clocks closer to earth, where the gravitational field lines are more concentrated, run slightly slower than those further away (e.g., clocks on mountaintops run slightly faster than those at sea level; GPS satellite onboard clocks run faster by about thirty-eight microseconds per day compared to clocks on the surface of the earth—thus they are adjusted for this gravitational time dilation effect). For simple problems like our marble | W PE curve, we often neglect these time effects in classical mechanics; when discussing the effects of massive objects on spacetime, it is common to focus on the curvature of space while neglecting the time dimension. This approach provides a reasonable approximation for many scenarios. That is, classical mechanics, which deals with potential energy curves like ours, often ignores relativistic effects. But it must be said that in extreme conditions (near black holes or at high velocities), both spatial and temporal components matter significantly. And not least precision instruments making precision measurements, when influenced by massive bodies, like the GPS systems mentioned, must account for both aspects. So, the W PE curve, as a function of space, captures the essence of classical mechanics just fine, and disregarding the time dimension of spacetime will facilitate our GR understanding of it and the marble’s behavior by way of simplification, but again, it is essential to recognize that spacetime curvature involves both components—space and time—and problem solving integrity lies in knowing when to approximate and when to dig deeper into the fabric of spacetime[2]. That said, let’s make an idealized and simplified GR right turn and see where it leads us and have some fun with it along the way. Imagine the W-shaped “curve” below as a possible representation of the local curvature of space(time) set up by the earth. The W shape would arise from the (spatial) interplay of tidal forces resulting from the variation in gravitational strength across the marble’s path, like so. When the marble moves closer to the earth, it experiences stronger gravitational attraction (lower potential energy). Conversely, moving away from the earth weakens the attraction (higher potential energy). These (spatial) variations create the characteristic “W” potential energy curvature (PE is a function of space | position, by definition). When disturbed, the marble would follow a deviated geodesic path in this quite peculiar W-curved space(time)—recall, matter and energy encode, very precisely, spacetime curvature, and this curvature in turn affects the motion of objects within it. Geodesics are the equivalent of straight lines in curved space (=shortest path in curved spacetime)—they represent the natural trajectories of objects influenced by “gravity.” (Aside, A. Einstein derived the geodesic equation by way of a least action principle computation like so: the actual path taken by a physical system between two points in spacetime is the one for which the action integral is minimized. The action integral is the integral of the pertinent Lagrangian (involves PE, KE, and in the context of geodesics, the metric tensor, and system coordinates as well) with differential dt, where t is the proper time experienced by the system “Creation Action”.) The marble follows a deviated geodesic path like so. Initially, the marble is at rest atop the W-shaped curve (maximum potential energy). When a disturbance happens, the marble’s (purely gravitational) geodesic path deviates—that is key against this backdrop: even an object initially at rest, as here, begins to “fall” along a geodesic in spacetime due to the curvature caused by massive objects (by “purely gravitational” is meant no other forces impinging on the system; the reader, sitting or standing somewhere on the surface of the earth while reading this, is falling along a purely gravitational geodesic by the way). Any influence that alters the marble’s motion from this purely gravitational “at rest” geodesic path constitutes a deviation in that path. As the marble now accelerates downward (deviates) it effectively “falls” along the deviated geodesic, following the peculiar (W) curvature of space(time) toward one or the other PE minima. And of course the marble’s potential energy decreases in lockstep as it falls along its deviated geodesic toward one of the minima, just as it would classically. So, in this idealization and simplification, the marble’s descent (geodesic-grooved fall) is a consequence of the space(time) curvature, and not a direct, classical gravitational force F acting on the marble. Potential is lowered (=transition to stability, that’s the key for our purposes in this study) but the dynamics are not strictly classical as related by the F in F=-del[V]. This equation is solid, and holds across the board in the created order, but we must rethink the potential-lowering F when dealing with certain gravitational problems: please notice, “F” is spacetime curvature in that context. We are going to show such marbles (W PE curve understood) as an example, but instead of marbles, we will look at grains of sand, which we will assume to be geometrically and physically ideal (hopefully this is the fun part). This example will try to show the key role that unstable equilibria serve in the created order—transition dynamics from stable equilibrium to unstable back to stable and so on, on a wide scale. We will show this via the discrete feedback dynamics of quite complex granular toppling and piling, decidedly a function of unstable equilibrium. In this short video, note that grains that are falling after passing through the slit are falling along their purely gravitational geodesic path (we are assuming no air resistance, wind, imperfections in shape, etc., that is, no deviation-causing outside influence—it is a purely gravitational geodesic path), and grains that are toppling throughout the pile and up in the grain box are falling along their impact-deviated geodesic path (Fig. 2).
Quantum Systems
Excited electrons in atoms “possess” excess energy, they are thus inherently unstable and quickly transition to lower energy levels. In short, it works like this. According to quantum theory, electrons in atoms occupy specific energy levels (quantized energy states) described by quantum numbers integral to our quantum computations (a lot of energy goings-on). When an electron absorbs energy, e.g., through the absorption of a photon, the absorbing electron becomes excited and moves to a higher energy level. (A photon is the fundamental “quantum,” i.e., “discrete lump” of electromagnetic energy; it is the carrier of electromagnetic interactions; it has energy in proportion to its frequency, it follows that said absorption is discrete; more properly said, a quantum is the smallest indivisible unit of a physical quantity such as energy). For our purposes, an excited electron is in unstable equilibrium in its excited state. To achieve stability, the electron spontaneously transitions back to a lower energy level, the lowest possible being the “ground state” (spontaneously, i.e., naturally, without external force or intervention—it is of course an inherent code). During this transition, the electron emits energy in the form of a photon (notice: photon absorption is a discrete “bumping up” of energy toward instability, and photon emission is a discrete “bumping down” of energy toward stability). The energy of the emitted photon corresponds precisely to the energy difference between the excited and transitioned to energy levels. The practical upshot is that the spontaneous, energetic transitioning down of excited (unstable) electrons serves as a crucial dynamic in the created order in that it drives overall functionality by way of light (energy) emissions needed further on down the causal pipeline, and by way of energy dissipation for thermodynamic functionality, and of course it is the main driver of chemical reactions (in the pipeline), and certainly not least it contributes to the aesthetic, colorful, amazing beauty of this world our Creator so lovingly made for us to live in and enjoy praised be His great Name.
Quantum entanglement
When dealing with electrons, the question of entanglement arises. Foremost for our purposes, quantum entanglement is not a form of unstable equilibrium; it is nevertheless worth a look in this study because in some instances the initiation of entanglement concerns a deeper interest of our study, namely the minimal energy configuration of systems is always preferred. What we are stressing is that the interplay of quantum systems seeking energy configuration minimization can lead to entanglement. Okay, entangled electrons (entangled particles per se) violate the principle of locality—they exhibit instantaneous correlations, regardless of distance, and this non-local behavior, together with their superpositioned state (until measured), and a general behavior that is governed by probabilities, rewrites our classical understanding of electron energy level transitioning to achieve stability as put forth above—essentially the electron (energy) absorption and emission mechanism discussed above takes on a new twist. The classical model of an electron’s absorption and emission mechanism (primarily the domain of atomic physics) does not fully hold for entangled electrons, in that regard quantum physics per se must guide the thinking, somewhat as follows. Entangled electrons achieve a form of stability through correlated behavior. When one entangled electron absorbs energy and becomes excited, the other adjusts (correlates) its state accordingly. Upon returning to the ground state, the excited electron emits a photon, (potentially) correlated with the other electron’s state—thus they step up and down by discrete lumps of energy…together—the “entangled electron polka,” a “waltz of wonder.” The synchronized stepping required of the dance partners here is mighty fast indeed—non-locality is their signature step. And their retractable holding arms in this dance, notice, they are apparently of infinite length.
Chemistry
Chemical reactions often involve transient states (=instability) that play important roles in reaction pathways, that is, in the reaction mechanism, itself the (successive) steps, at the molecular level, that occur during a chemical reaction. These steps govern how reactants transform into products. The red font text is “chemistry in a nutshell.” That is, the rearrangement of atoms and molecules to form new substances lies at the heart of chemistry, be it a simple combustion reaction or a complex organic synthesis—the heart of chemistry lies precisely in these transformative steps. For example, during combustion, free radicals (anything with an unpaired valence electron—highly reactive) serve as unstable intermediates before forming stable products. In that regard, consider the combustion of methane, wherein hydroxyl and methyl radicals are formed as intermediates. As the name suggests, intermediates are transition states between reactants and final products—they are species that form during the reaction, they are neither the reactant, nor the final product, and they exist only temporarily. They are unstable in the sense of high reactivity and attendant short lifetime/fast decomposition. But in the course of their short lifetime, they drive the reaction; they facilitate bond-breaking/forming, and they influence reaction rates and selectivity. Again, notice the dynamics, the transition point centered on an unstable equilibrium, and not least the impact on overall functionality (product formation in this instance). We see in this interplay between stable and unstable equilibrium the design and scheme of a genius to put it mildly.
Economics
Financial markets can and often do manifest unstable equilibria. For example, consider a stock market “bubble.” When prices rise rapidly due to crazed speculative buying, it creates an unstable equilibrium, i.e., a potential transition point as mentioned above. A small shock (a downturn in sector/s earnings, unexpected federal reserve action, etc.) can trigger a collapse, that is, a market “correction” (no restoring force implied). These corrections, once past the “tipping point,” i.e., maximum tolerable instability (sort of like a maximum PE), are abrupt and vicious (hyperbolic; unstable equilibria whatever the setting typically “correct” hyperbolically). Similarly, economic policies per se can create unstable conditions. For example, maintaining low interest rates for an extended period may create another sort of “bubble,” asset bubbles, which eventually “burst” (again, hyperbolically). These transitions lead to a reshuffling of wealth, and so it goes, thus cycling back and forth and back again from financial stability to instability back to stability all the while reshuffling wealth—therein lies the functionality.
Meteorology
The weather, one of our Lord’s (global and local) game changers, exhibits unstable behavior. For example, the onset of thunderstorms involves unstable air masses rising and condensing. Yet thunderstorms are crucial for our planet’s sustained water supply. ( Note 1Kings 18:41-44—here we see God’s sovereignty, His ability to bring rain, to end a severe drought, and His care for His faithful servant, Elijah. It is a powerful reminder that God is in control, even in the midst of challenging circumstances.) Moreover, we benefit from the lightning strikes:
(lightning+N2)–>N+O2–>(NO,NO2)+rain water–>(HNO3)+water vapor–>(NO3– nitrates).
The lightning strikes, while dangerous, release nitrates from the encoded atmospheric reactions shown above. These strikes are thus “natural fertilizers,” responsible for some twenty percent of the nitrogen in soil. (The water, the nitrates, just a couple of examples of the functionality this unstable equilibrium manifests, there are far more than that.) Another one of our favorites in this category is the ice-albedo feedback loop (negative feedback) which feedback is literally modulated by unstable equilibria. Isn’t it marvelous how our Creator God does things dearly Beloved? So elegant (=efficacious simplicity) , so pure, i.e., unfeigned, so lovely, all His handiwork…
Our Soul
We saved the most important one for last. Sin is unstable equilibrium. Where (who) is the restoring force? This unstable equilibrium (the Sin bent) is missing a very elegant, and pure, and lovely Restoring Force indeed—it need not remain unstable forever for you and me beloved reader, there is possible a transition point unto holiness, the holiness stability of Jesus Christ imparted to His Beloved, with attendant eternal life in the presence of very God (”A Letter of Invitation”)
Concluding Comments
In this study our goal was to dig deeper, beyond first glances, to appreciate the role of unstable equilibria in the created order. An unstable equilibrium is one that is very delicately “balanced” with respect to any number of free variables, and which can quite easily “tip over” to an “unbalanced” state. At first glance they seem to be out of place in a Creation manifested by a God of order and stability, but peeling back the layers clearly shows their crucial role in attaining the very functionality intended by our Creator. They mark transition points unto stability, and in this way, they shoulder the dynamics quite assumed in said functionality. Imagine a universe without them—it would be stagnant, static, and “stuck.” Nothing could mature. There would be no need for chemistry, or physics, or the mathematics that undergirds these disciplines. There would be no causals, no colors, no curves, a pretty boring place. That is not how our Creator does things, no way (it is not in keeping with His self-revelation through His Word). So, He interjected them here and there and everywhere and O my immediately boring became exciting. Immediately we see His chemistry, and physics, and mathematics, and practical engineering. From boring to exciting in one brilliant stroke, just by interjecting them into His creative handiwork. (And please notice, the second law of thermodynamics recognizes this need for unstable equilibria; it implies that any given state of equilibrium is likely to be unstable.) And the stable equilibria work in the other direction, not allowing the unstable equilibria to drive the created order into irrecoverable chaos, i.e., “stuck” in the other direction. The genius in it all is clearly how these two equilibria are designed to achieve harmonious functionality “on the fly” through their interplay, at all scales (quantum on out to relativistic)—that is no easy task and bespeaks genius in the extreme. So, while stable equilibria provide balance, unstable equilibria lend to the dynamism and adaptability of systems in the created order. They allow for transitions, maturation, and resilience. The two “shake hands” beautifully, coming together in space and time to manifest an aesthetic and exciting and pleasing reality.
And so it is in the created order, but there is a more aesthetic and exciting and pleasing reality that concerns our souls. It is Salvation. Recovery from the unstable equilibrium of Sin by the Restoring Force of God. This reality is serious, and stark, it is Salvation-serious and stark. It is separation-sentence stark, indeed, Salvation restores our right relationship with Righteous God, even on into eternity. Beloved reader, much precious in the eyes of your Creator God, please don’t miss out on that restoration, even Salvation (“A Letter of Invitation”).
Praised be your Name great Creator God, whom we love and adore. Amen.
Illustrations and Tables
Figure 1. Arbitrary PE Curve.
(divide by marble mass=V Curve)
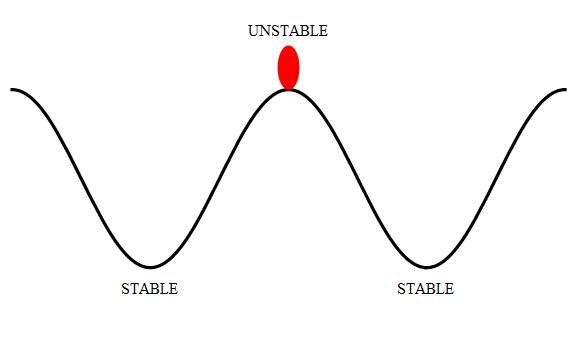
Figure 2. Granular Toppling and Piling.
(slit width=1 grain)
Works Cited and References
“A Letter of Invitation.” (A standing invitation.)
Jesus, Amen.
< https://development.jesusamen.org/a-letter-of-invitation-2/ >
Dewus, Michael.
“Granular Toppling and Piling.”
Online Publication (February 2012)
< https://demonstrations.wolfram.com >
Gentry, Robert V.
Creation’s Tiny Mystery.
Knoxville: Earth Science Associates 1992.
Microsoft Copilot (March, 2024).
Notes
[1] The classical perspective of the marble’s response to a disturbance is as follows. The marble starts at the unstable equilibrium position atop the middle peak of the W. When gently nudged and therefore linearly (not wildly) displaced, it does not lose contact with the W throughout its descent. As the marble rolls down into one of the valleys, its spatial location energy (PE) decreases while its kinetic energy, i.e., motion energy (KE), increases. At the valley bottom, KE is maximized, and PE is minimized. Due to its kinetic energy, the marble tries to climb up one of the side walls. However, it can never reach a height higher than the original peak because that would imply gaining energy from somewhere, which violates the Creator’s principle of conservation of energy—the marble’s total energy must remain constant throughout, and so it transitions between PE and KE, with total energy at any time being PE+KE. Initially, before the nudge, the marble’s PE is maximum, and its KE is zero, after the nudge, and descent into one of the valleys, its PE is zero and KE is maximum, and at all times in between we have some amount of PE and KE that sums in such a way as to keep the marble’s total energy constant—and that total energy is precisely the PE the marble had initially—that amount of energy is maintained throughout. Eventually, the marble settles into a stable equilibrium position within one of the valleys. Here, its potential energy is approaching the minimum and it has enough kinetic energy to oscillate back and forth within the valley for a while but without ever surpassing the initial middle peak height. The marble will lose its total energy to friction and thermal effects and become stationary at the bottom of the valley in the end, and that energy loss is gained by the universe as an overall increase in its entropy—it is a zero sum game from the Creator’s perspective from first to last—the universe’s overall energy (imparted by Him) remains constant in this way, in keeping with the first law of thermodynamics. Not one jot or tittle of His precious energy is ever lost. Please notice, the conservation of energy is a fundamental principle that encompasses the first law of thermodynamics. Both emphasize the constancy of total energy, whether in thermodynamic systems or across the universe. So, we see that the principle of conservation of energy extends beyond thermodynamics (it is broad) and asserts that the total energy in any isolated system remains constant. Energy can transform from one form to another, e.g., kinetic to potential, thermal to mechanical, but the total amount remains unchanged. Well and good as far as all that goes, but more specifically concerning our marble, what provides the restoring force that maintains stable equilibrium in a given PE minimum—recall that was integral to our definition of stable equilibrium? For starters, let’s consider the obvious. As the marble climbs a side moving away from the stable equilibrium, which is at the very bottom of the valley, gravity pulls it back down. But why? The marble’s gravitational potential increases as it climbs, and gravity provides the F in F=-del[V] thus lowering its potential (this potential lowering behavior is a given throughout the created order). But we must go a step further by considering the sides that lend to a given valley’s overall “U” shape, we must consider more purely lateral forces (“gravity” is shouldering the vertical restoration). One component of lateral restoration is a contact “push back” force (called a “normal” force, normal as in perpendicular; normal forces play a crucial role in maintaining stable equilibrium; when two objects come into contact, a normal force comes about because of interactions at the atomic level between the molecules of their surfaces, it prevents solid objects from “passing through” each other and it always acts perpendicular to the contacted surface, i.e., it pushes away from that surface, opposing any force that tries to compress or penetrate the objects [not to be confused with degeneracy pressure, which is a quantum effect]). Here is why we spent some extra time discussing the normal force. When the marble rolls along an arc of the U-shaped bottom, it tries to undergo uniform circular motion, which requires a centripetal force as mentioned above, and said normal force (“push back force”) provides that centripetal force which acts as a lateral restoration (more like a multi-angled constraint). Now imagine the sides as being “springy,” i.e., elastic. When the marble moves away from a valley bottom and up a given side, that side deforms elastically if ever so slightly, in turn the deformation stores elastic potential energy in that side itself, and that energy, mediated by the deformed side, exerts a lateral restoring force by way of doing work, thus bringing the marble back toward stable equilibrium. Work is energy, elastic potential in this instance, it is, in this instance, [restoring] force times [deformation] distance, all alike mediated by the deformed side. This force opposes any lateral deviation from the stable equilibrium position. (When a surface is deformed, the direction of the normal force adjusts to be perpendicular to the new surface shape.) That is how the marble’s lateral stabilizing restoring force manifests looking at it from an energy perspective. Hooke’s Law can quantify this lateral restoring force more readily for us:
Frestore=-k x,
where k is the “spring,” or deformation constant of the sides (a function of resistance to deformation), and x is the lateral deformation distance. The minus sign indicates that the force direction is opposite to the deformation direction. If these parameters can be determined, then we can quantify the restoring force. But we are talking about an intangible PE curve here, so we must leave it unquantified and appreciate it qualitatively by way of a thought exercise as attempted here. So, gravity (vertical restoration), and two manner of lateral forces, one a centripetal “push back” force, and another an elastic Hooke’s law force are, by working together, providing the restoring force that tries to keep the marble in its stable equilibrium.
[2] Newtonian gravity is our trusty workhorse for everyday (classical) problems, but it is an incomplete theory; while it serves us well, it has limitations that become apparent already in moderate gravitational conditions as posed by our earth. Consider the matter of “cosmic precision” (a technical term utilized in the field of cosmology signifying precise measurements of cosmological parameters and properties such as cosmic microwave background [CMB], redshifts, et al.). GR is our workhorse to achieve cosmic precision, as exemplified by the following abbreviated list of scenarios GR satisfactorily addresses. When launching satellites or spacecraft, precise calculations are essential because even tiny errors can lead to significant deviations in trajectories. The Global Positioning System (GPS) mentioned before relies on cosmic precision: the satellites’ positions and clocks must be known with great accuracy to provide reliable navigation. (Massive objects like earth cause a gravitational redshift in light. When signals from satellites travel through earth’s gravitational field, they experience this redshift. GPS satellites emit signals at specific frequencies, and due to gravitational effects, these signals appear redder (lower frequency) when received on Earth.) The study of distant stars, galaxies, and black holes requires precise measurements of their distances, luminosities, and positions. And not least measuring the expansion rate of the universe demands cosmic precision.